Reflection in triangle 2018

03/17/2018 បញ្ចេញមតិ

2018

Let P_0, Q_0, R_0 be points lies on B_0C_0, C_0A_0, A_0B_0.

Let P_k\in [A_0P_0) such that A_0P_0=P_0P_1=...=P_{k-1}P_k and Let A_k\in [P_0A_0) such that P_0A_0=A_0A_1=...=A_{k-1}A_k.

The same ways we get Q_k, B_k and R_k, C_k.

Prove that \displaystyle \frac{[A_kB_kC_k]-[P_kQ_kR_k]}{[A_0B_0C_0]-[P_0Q_0R_0]}=2k+1

Author: Van Khea, Cambodia

Filed under Uncategorized

Problem 14/01/18

01/14/2018 បញ្ចេញមតិ

p14

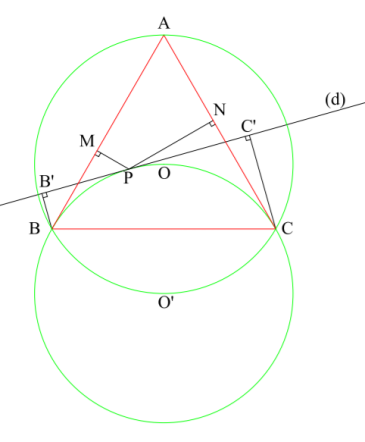
Let O be circumcenter of an equilateral triangle \Delta ABC and let O' be circumcenter of \Delta BOC. Let (d) tangent to (O') at P. M, N are projection points from P to AB, AC. B', C' are projection points from B, C to (d).

Prove that PM+CC'=PN+BB'

Author: Van Khea, Cambodia

Filed under Uncategorized

P17, equilateral triangle

12/17/2017 បញ្ចេញមតិ

p17

Let D be point lie on arc BC of an equilateral triangle \Delta ABC  such that \angle DAC=15^0. Let E and F be points such BE//AD and CF//AD.

Prove that AD^2+BE^2=2.CF^2

Author: Van Khea, Cambodia

Filed under Uncategorized

Problem 02 (An Equilateral triangle)

12/10/2017 បញ្ចេញមតិ

10

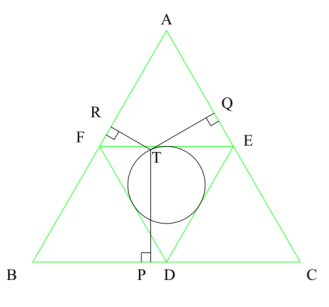
D, E, F be midpoints of an equilateral triangle \Delta ABC. T be point lie on incircle of \Delta DEF.

Prove that \displaystyle BP+CQ+AR=\frac{3}{2}.AB

Author: Van Khea, Cambodia

Filed under Uncategorized

Problem 08

12/08/2017 បញ្ចេញមតិ

08

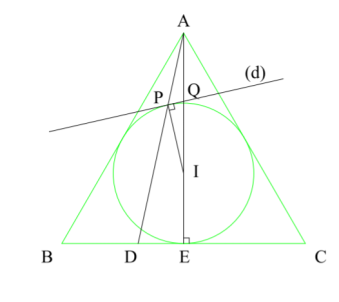
Line (d) tangent to incircle of an equilateral triangle \Delta ABC at P.

Prove that \displaystyle \frac{AP}{PD}+\frac{AQ}{QE}=1

Author: Van Khea, Cambodia

Filed under Uncategorized

Line tangent to circumcircle

11/25/2017 បញ្ចេញមតិ

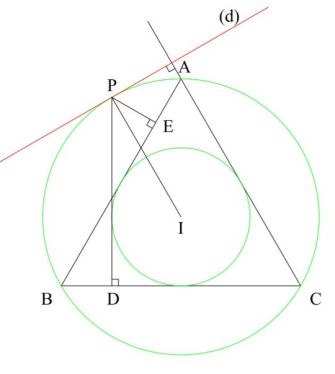
problem

\Delta ABC is an equilateral triangle. Prove that:

1) PD=PI+PE

2) \displaystyle PD^4+PE^4+PI^4=\frac{1}{2}.AB^4

Author: Van Khea, Cambodia

Filed under Uncategorized

right triangle inequality

10/18/2017 បញ្ចេញមតិ

c1

Let \displaystyle \frac{AH}{HC}=\frac{7}{3}.

Prove that \displaystyle AB^3+BC^3<\frac{3}{4}.AC^3

Author: Van Khea, Cambodia

Filed under Uncategorized

← អត្ថបទ​ចាស់​ជាង​នេះ​